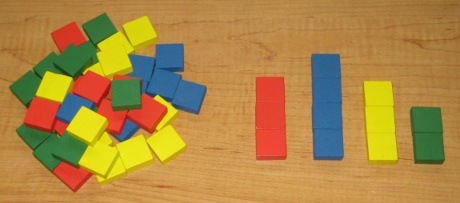
We’ve enjoyed lots of games and activities with one inch wooden color tiles and cubes. Here are a few of the highlights.
Scoop and Sort: Scoop a handful of tiles and sort them into various piles, columns, or groupings of colors. How many of each color do you have?
Filling Boxes: It’s fun to fill small shallow boxes with various patterns, colors, and numbers of tiles.

- How many ways can you play with the idea of one half?

- How many tiles of each color do you see in each box? Do you see a box with 4 yellow and 5 red? Do you see a box with 2 out of 6 parts yellow? How about 3 out of 9 parts red?
Square Fill-in Puzzles: How many different ways can you fill in the squares? What combination of tiles will you choose?
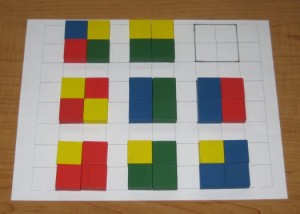
- How many different ways can you fill in the 2 by 2 squares?
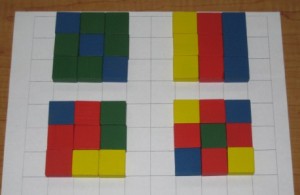
- How many different ways can you fill in the 3 by 3 squares?
Roll a Rectangle: Partners take turns rolling a set of dice. Together, arrange color tiles into rectangles according to the numbers on the dice. For example, if you roll a 3 and a 4, then build a 3 by 4 rectangle; if you roll a 2 and a 5, then build a 2 by 5 rectangle. How many tiles are in each rectangle? How many different rectangles you can build?
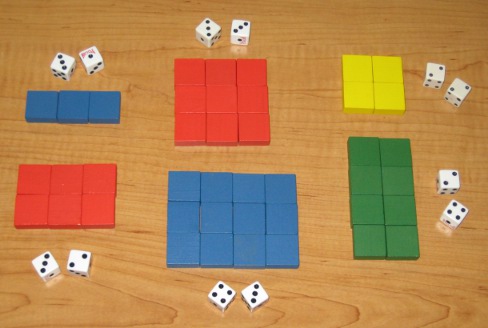
- Dice Game: Roll a Rectangle
Spaghetti and Meatballs for All! by Marilyn Burns
This is a fun story about a woman who used 8 tables to seat 32 people for a big dinner gathering. As people arrived, they kept pushing the tables together and moving them around in different configurations which caused many problems related to the total number of people who could be seated.

- If you think of these sets of tiles as tables, how many people can sit at these various table arrangements? (Just one person per side.) Which arrangement will seat the most people? Which will seat the least?
Pentominoes: Create a variety of pentomino shapes with five square tiles.
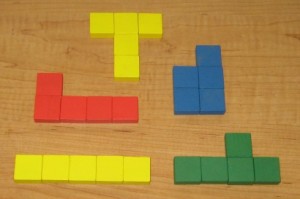
- How many different pentomino shapes can you build?
10×10 Grid Designs: Create colorful patterned designs on a 10 x 10 grid.

- How many yellow tiles out of 100? How many red or green? How many are blank?
Building Up with Color Cubes: Color cubes are fun for building pyramids, towers, and other 3D creations.
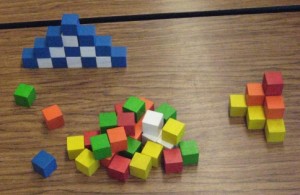
- One inch color cubes
Tags: color tiles, mathematics, play
Category Square Color Tiles, What Do You Do All Day? |

- Guitar headstock built by Don Miller
Simple conversations are the heart of family life. Here’s one I overheard recently:
Don came in from the woodshop to talk to Ben while Ben was relaxing in the living room. Don asked, “Do you have any rosewood?”
It struck me as a funny question for a dad to ask a son, but as they continued, I realized they were talking about guitar woods. They talked about the rosewood Ben found last year, what Don might use it for, and other related details. The exchange lasted only a few minutes, but it’s one of many that keeps them connected. I enjoyed overhearing this unique conversation between them. It reminds me how nice it is that they have time to share materials and talk about projects together.
Conversations don’t have to be big or important to be valuable. Even the simple ones can help us appreciate each other, rely on each other, and work together.
Tags: conversations, projects, what do you do all day?
Category Projects, What Do You Do All Day? |

- How many apples does it take to make a pie? How long does it take to bake? At what temperature? When you slice it, how many pieces will you have?
When you’re walking around in your everyday life, do you see the mathematics all around you? Do you notice shapes, sizes, angles, and patterns? Do you see the potential for counting and sorting and comparing items? Not as lessons, but just as cool things to observe as you’re going about your business. If you notice these interesting parts of life, and enjoy the math all around you, your kids will too. Your lives will be full of patterns and numbers, puzzles and games, and fascinating problem-solving experiences every day.
One year, we bought a large box of pears for canning. Ben took them out of the box and laid them out ever-so-carefully on the table, in a spiral pattern. He started in the middle of the table and then circled them around each other, spiraling outward until he reached the edges of the table. Then he counted them to see how many there were. Just because.
When he turned 8 years old, Ben exclaimed, “I’m an even number again!” I’ve always loved remembering that. It was a celebration of who he was, as well as a simple observation about the numbers in his life. He’s more than twice that age now, but he still notices numbers and patterns like that.
If you see the math all around you, then you can appreciate it as a natural part of your life. It’s something that’s useful and ordinary and amazing all at the same time.

- When you see this garlic braid, do you count 1, 2, 3, 4, 5, 6, 7, 8, 9 garlic bulbs? Or groupings of 3, 6, 9? Or maybe you think, how many rows of garlic will I need to plant so we can make several garlic braids next year?

- When you see these fruits and veggies, do you think about shapes or colors or numbers? Do you count them or think about their groupings? Do you notice odd and even numbers? Do you wonder how long it is till lunch?

- How many longboards can you build in a month? How many trips to the lumber store will it take? Which shapes do you like best? How many layers of 1/4 inch plywood will you need? How much will you spend? How much pride and happiness is on his face?

- How many wall grips do you need when building a climbing wall? How far apart should they be? Should you lay them out systematically on a grid, or randomly? How much weight will they hold? What are the best angles for a climbing wall?

- If you see it snowing at night, do you wonder how much snow there will be in the morning? Enough for sledding? And what's the temperature outside?

- How many pumpkins and how many of each size and variety? Do we have enough to keep for winter and some to give away?
Tags: connections, creating our own path, mathematics, play, problem solving, what do you do all day?
Category Connections, Creating Our Own Path, For the Love of Math, What Do You Do All Day? |

The topic of classes, camps, programs, and workshops for young or new unschoolers came up on a discussion list this week. It got me thinking about the variety of adventures we’ve enjoyed over the years, and how we went about choosing those we participated in. It also reminded me how we weren’t quick to sign up for every class or program that came along. During the discussion, the idea was raised that it’s important for unschoolers to give themselves adequate time and space away from feeling the need for academically focused classes and programs so that they have time to really see and appreciate the natural, everyday learning all around them. I thought it was an important discussion, so I’m bringing some of the details and my comments here.
This is a comment from Sandra that sparked my own response:
“…if beginners don’t go through a phase in which they REALLY focus on seeing learning outside of academic formalities, they will not be able to see around academics. If you turn away from the academics and truly, really, calmly and fully believe that there is a world that doesn’t revolve around or even require or even benefit from academic traditions, *then* after a while you can see academics (research into education, or classes, or college) from another perspective.”
My response:
For the longest time, some of our homeschooling and unschooling friends didn’t really understand why we didn’t always sign up for the latest and greatest offerings of classes or programs available in our community: the homeschool science co-op with the really fun teacher, the full-time local alternative school programs directed at homeschooling families, etc.
We didn’t feel that we needed them, and we didn’t want our lives constrained by endless schedules or the implications of such structured learning situations when Ben was young. He wasn’t that interested, and we knew that there was plenty to explore naturally on our own.
I think because we chose things very carefully early on, it helped us retain our independent thinking and mindfulness about learning from ordinary situations instead of feeling the need for formally structured situations. We knew Ben could learn naturally by going places and doing things with friends and family. It was more interesting and meaningful to go to the zoo or aquarium, or explore the woods and garden out back, or play in the park with friends than to sign up for early science classes or other lessons and formalized activities.

This photo is from our weekly lake days with friends. We went to the same lake to swim, picnic, and relax together every Friday of every summer for at least a decade. Ben (2nd from the left) was about four years old. He’s still close with a few of these friends now.
Sometimes it’s hard to pass up programs that everyone else is involved in, but it’s easier when you’re clear about what you want or are interested in. Ben was usually pretty clear about activities that were important to him and those that weren’t. Over the course of a dozen years, he enjoyed some gymnastics, a unicycling club, a few metalworking classes, and a wilderness program. When he was 15, he was offered a job helping at a ropes course, but first he needed a first aid/CPR certification. So he took a class. This was the first remotely academically oriented class he had ever taken, and he did great! He was ready. He didn’t need anything like it before, and then he did. It was fine.
Today, at 17, he’s taking an introductory fire service technology class for a couple hours a day — mainly because another friend had the year before and talked it up so much, and it sounded like a cool opportunity. It’s part in-class-academic work and part outdoor hands-on training. He’s really enjoying it. He may or may not choose to pursue firefighting in the future. It’s just something to explore now — one opportunity among many in a rich life.

About ten years later, swimming with friends on a camping trip.

Tags: adventures, creating our own path, growing up, what do you do all day?
Category Adventures, Creating Our Own Path, Growing Up, What Do You Do All Day? |

Yesterday, while Ben was reading a book called Born to Run, he read something about molasses, about a runner who liked using molasses instead of other sweeteners. We talked about it briefly, about various sweeteners, how people’s tastes have changed over time, and that was about all.
Then this morning, Ben said he had looked up “molasses” to read more about it, and at the same time, he found an article about the Boston Molasses Disaster, also known as The Great Molasses Flood of 1919. I had never heard of it before. He showed me the article, and we laughed at the absurdity of how it sounded, but then also took note of the tragedy involved since 21 people had died and 150 were injured. A fifty foot molasses storage tank had ruptured and collapsed, flooding a couple million gallons of crude molasses into the streets of Boston’s industrial North End at an estimated 35 mph. Amazing!
We talked about it a bit more, still amazed at such an odd disaster, and amused that he ran across this story just because he wanted to know more about molasses. I think Ben summed it up well: “That’s the funny stuff you find out when you look up something ordinary.”
So true. One thing leads to another, and you learn something new every day.

- The Great Molasses Flood: Aftermath of the disaster; photo by Globe Newspaper Co. (Boston Public Library)
A few more details, if you’re curious:
The Great Boston Molasses Flood
Molasses Clocked at 35 MPH…in January!
Dark Tide: The Great Boston Molasses Flood of 1919
Tags: connections, conversations, reading, what do you do all day?
Category Connections, Creating Our Own Path, Reading, What Do You Do All Day? |

Geoboards come in a variety of sizes and patterned grids, and they’re lots of fun to play with using an assortment of colorful rubber bands. You can create repetitive designs, six-pointed stars, triangles, squares, hexagons, rectangles, and all kinds of other shapes.
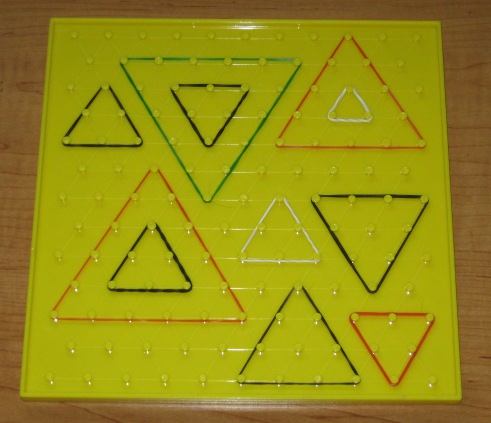
- Triangles and more triangles

- Hexagons within hexagons

- It's fun to copy shapes with a partner, making your shapes exactly the same, or larger or smaller.

- How many triangles do you see in the six-pointed stars? More than two? Do you see any hexagons? How many segments do you see in each of the other designs?

- Squares are fun to build in a variety of sizes: 1x1, 2x2, 3x3, 4x4, 5x5

- Square color tiles and geoboards are great for playing with together. The color tiles can make it easier to see the number of individual squares within a given shape.
Tags: art, geoboards, mathematics, play, toys & tools
Category Art, For the Love of Math, Geoboards |
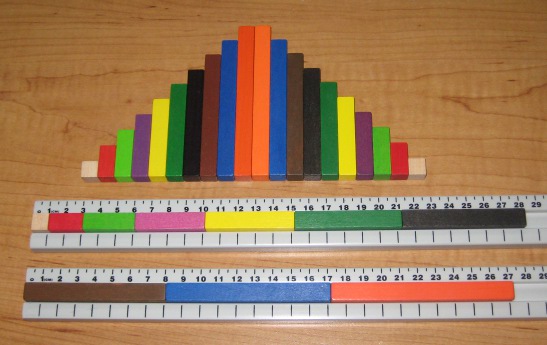
- Cuisenaire rod staircases and rod trains in rod tracks
Cuisenaire rods are colorful wooden rods ranging from 1cm to 10cm lengths. They’re great for creating patterns, counting, sorting, measuring, adding, multiplying, and all sorts of other fun explorations. Although the rods were originally created for formalized classroom use, as a curriculum component, I hope you choose to just play with them instead. I think your experiences with them will be more enjoyable and longer lasting that way. If you treat them like workbooks and assignments, it may detract from the intrinsic fun and natural learning that’s possible. The following are some of the ways we’ve played with them over the years. Enjoy!
Creating Patterns and Designs
- Use the rods to create patterned designs. Experiment with symmetrical and asymmetrical patterns.
- Think about the colors and sizes of the rods first rather than the numbers.
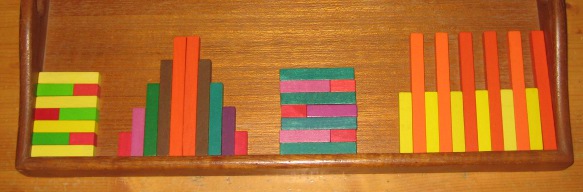
- A few Cuisenaire rod patterns
Yellow = green + red = red + green (5 = 3+2 = 2+3)
Red, purple, green, brown, orange, orange, brown, green, purple, red (2,4,6,8,10,10,8,6,4,2)
Dark green = purple+red = red+purple (6 = 4+2 = 2+4)
Yellow, orange, yellow, orange (5,10,5,10)

- Expanding Cuisenaire rod rectangles
Building Trains and Staircases
- Use the rods alone, or the rods and tracks, to create varying lengths of trains.
- Use all one color rod, two colors together, or a combination of colored rods for different trains.
- Use the 50cm or 100cm tracks to measure the trains easily.
- Use dice to add or multiply numbers to determine how long of a train to create.
- Use rods to create varying heights of staircases, maybe with a difference of one or a difference of two in each step. See the first couple photos above.

- 12 reds is the same as 8 light greens is the same as 6 purples is the same as 4 dark greens is the same as 3 browns
Dice Games with Rods & Tracks
These are fun games to play with a partner, and a great way to familiarize yourself with the rod colors and their corresponding number values. You can play these games with either 50cm or 100cm rod tracks, a set of Cuisenaire rods, and a set of dice.
Race to 50 or Race to 100: Each player takes a turn rolling a die to see which rod to place on his or her track. For instance, if you roll a 5, you can use the metric ruler on the edge of the rod track to measure the rod you think represents the number 5. You might have to measure a few rods at first to know which one is the right size. Each player takes turns placing corresponding rods on his or her track. After each turn, you can compare the lengths of rod trains on your tracks. Play until one or both players reach the end of the 50cm track.
Variations: You can use one die, placing a single rod at a time on the track, or a set of dice to either add or multiply the numbers on the dice. If you’re adding the dice, and you roll a 2 (red rod) and a 3 (light green rod), you can choose to place the red and light green rods or a yellow rod (5) on your track. If you’re multiplying the dice, and you roll a 3 (light green) and a four (purple), that makes 3 fours or 4 threes, so you can place 3 purples or 4 light greens on your track.
Race to Zero: This is the reverse game of Race to 50 or 100. Fill up a track with orange rods (10s), roll the die, and subtract rods till you clear the track and get to zero. Trade rods as necessary in order to facilitate subtraction. This is a game that is best played after many games of Race to 50 or 100 because subtraction concepts can be more abstract to think about while becoming familiar with the rods and tracks. Alternately, you could just keep the original rods from a Race to 50 game on the rod tracks, and remove one colored rod at a time according to the number you roll. In this way, there’s no trading involved, just identifying the numbers/colored rods to remove.

- Race To 50: At this point in the game, one player has reached 28cm and the other has reached 30cm.
Game: Half Of…
This is a simple conversational game for young children. It’s adapted from the book, First Grade Diary, p.4 by Robert Hightower and Lore Rasmussen.
- Can you find the rod that is “half of” the purple rod? (The red rod is half the length of the purple rod.)
- Can you find “half of” the red rod? Which rod is “half of” the orange rod? Etc.

- Light green is half of dark green; red is half of purple; purple is half of brown; white is half of red; yellow is half of orange
Creating Squares
Can you find some “square numbers” by creating Cuisenaire rod squares with 4 fours (purples), 5 fives (yellows), or 6 sixes (greens)? If you then shift them into lines on a rod track, you can confirm their totals. For example, a 4×4 = 16, a 5×5 = 25, and a 6×6 = 36.
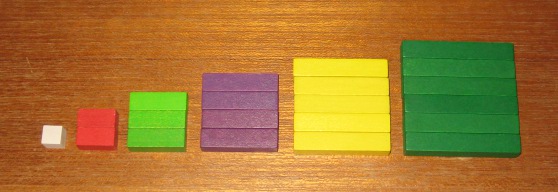
- 1x1, 2x2, 3x3, 4x4, 5x5, 6x6
Game: How Long, How Many…
Use centimeter graph paper and some Cuisenaire rods to play this game of building rectangles. Roll a set of dice. The 1st roll tells how long a rod to use; the 2nd roll tells how many of that colored rod to take. Arrange the rods into a rectangle. You can place the rod rectangles on a sheet of centimeter graph paper, or also trace them on the paper and write the related multiplication sentence inside each rectangle. (For example, 3×5=15, or 4×6= 24) Play until the graph paper is covered with as many rod rectangles as possible. This game is adapted from the book, About Teaching Mathematics: A K-8 Resource, 3rd Edition, p.201 by Marilyn Burns.

- How Long, How Many: 4 sixes (dark greens), 2 fives (yellows), 5 threes (light greens), 4 fours (purples), 3 twos (reds)

- How Long, How Many: One way to play this game is after you place the rod rectangles on the paper, such as 4 of the sixes (dark greens), you can trace the rectangles and label them (4 of 6, or 4 x 6).
Parts of a Whole
I think Cuisenaire rods are helpful when thinking about parts of a whole. Kids will notice these relationships naturally, but I’ve mentioned them here so parents can see and think about them also. For instance:
- If an orange rod is considered one whole, then a yellow rod is one of two parts, or ½ of an orange rod.
- If a brown rod is one whole, then a red rod is one of four parts, or 1/4 of a brown rod.
- If a blue rod is considered one whole, then a light green rod is one of three parts, or 1/3 of a blue rod.

- Illustrating parts of a whole
Logic Problems
These are simple games of logic using Cuisenaire rods. Hide a small collection of rods and offer clues to help people predict which rods you’re hiding. Participants should have a small assortment of rods in front of them to help them visualize the clues and make good predictions. For example:
- “I have three rods. They’re all different. The train is as long as dark green.” (light green, red, white)
- “I have four rods. All are the same. The train equals orange plus dark green.” (four purple)
- “I have four rods. None are the same. The train equals three yellow minus purple.”
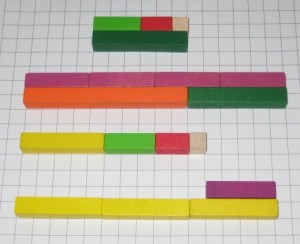
There are numerous problems like these from the book, Hidden Rods, Hidden Numbers by Manon Charbonneau. It was re-published with a new title, Critical Thinking Puzzles with Cuisenaire Rods. I think they’re both out of print now, but you might be able to find a used copy. I found my copy of the first title many years ago at Math ‘n’ Stuff in Seattle. Of course, you can always just make up your own similar guessing games. Be sure to let your kids take a turn choosing the rods and clues, too.
Have fun playing!
Tags: art, cuisenaire rods, games, mathematics, play, toys & tools
Category Art, Cuisenaire Rods, For the Love of Math |
 Over the years, we enjoyed a wonderful collection of children’s fairy tales. We read traditional tales, newer versions from favorite authors, and variants from different countries. The more we read, the more we were able to find various retellings, often with plot twists or character differences that added new charm to the old stories. Some were humorous, some were written in verse, some had amazing illustrations, and some told the stories beautifully even as wordless picture books.
Over the years, we enjoyed a wonderful collection of children’s fairy tales. We read traditional tales, newer versions from favorite authors, and variants from different countries. The more we read, the more we were able to find various retellings, often with plot twists or character differences that added new charm to the old stories. Some were humorous, some were written in verse, some had amazing illustrations, and some told the stories beautifully even as wordless picture books.
The following is a list of some of the fairy tales that Ben and I enjoyed reading together when he was younger. I also read many of these picture books with my students while teaching fourth and sixth grades, oh so long ago. Even though they are often written for a younger audience, many are delightful retellings that older children really appreciate. Some of the variants, such as those by Roald Dahl and Allan and Janet Ahlberg, are especially fun for children who are quite familiar with the traditional tales.
Most of these books you’ll be able to find in your local library, which of course will lead you to more and more fairy tales, depending on your library’s catalog. If you browse the titles online, you’ll also be able to read a bit about each version as well as find other similar titles. Happy reading!
The Three Little Pigs by Paul Galdone
The Three Little Pigs: An Old Story by Margot Zemach
The Three Little Pigs Retold and illustrated by James Marshall
The True Story of the Three Little Pigs! By A. Wolf As told to Jon Scieszka, Illustrated by Lane Smith
The Three Little Wolves and the Big Bad Pig by Eugene Trivizas, Illustrated by Helen Oxenbury
Red Riding Hood Retold and illustrated by James Marshall
Little Red Riding Hood Retold and illustrated by Trina Schart Hyman
Red Riding Hood Retold in verse by Beatrice Schenk de Regniers, Drawings by Edward Gorey
Lon Po Po: A Red Riding Story from China by Ed Young
Goldilocks and the Three Bears Retold and illustrated by Jan Brett
Goldilocks and the Three Bears Retold and illustrated by James Marshall
Deep in the Forest by Brinton Turkle
Alaska’s Three Bears by Shelley Gill, Illustrated by Shannon Cartwright
Jack and the Beanstalk Retold in verse for boys & girls to read by themselves by Beatrice Schenk de Regniers, Illustrated by Anne Wilsdorf
Jim and the Beanstalk by Raymond Briggs
Hansell and Gretel Retold and illustrated by James Marshall
Cinderella Retold by Amy Ehrlich, Illustrated by Susan Jeffers
Yeh-Shen: A Cinderella Story from China Retold by Ai-Ling Louie, Illustrated by Ed Young
Princess Furball Retold by Charlotte Huck, Illustrated by Anita Lobel
The Paper Bag Princess Story by Robert Munsch, Art by Micheal Martchenko
Princess Smartypants by Babette Cole
Thorn Rose, or The Sleeping Beauty by The Brothers Grimm, Pictures by Errol Le Cain
Snow White in New York by Fiona French
Rapunzel Retold and illustrated by Paul O. Zelinsky
Rumpelstiltskin Retold and illustrated by Paul O. Zelinsky
The Frog Prince Continued Story by Jon Scieszka, Paintings by Steve Johnson
The Jolly Postman or Other People’s Letters by Allan and Janet Ahlberg
Roald Dahl’s Revolting Rhymes With full-color illustrations by Quentin Blake
* * * * * * * * * *
If you search for the phrase “fairy tale variants” or “variations,” you’ll find plenty of great lists like these:
Fairy Tale Variants
Fairy Tales: A bibiography of popular stories
Folk Tales and Fairy Tales: Retellings, Adaptations, Variants, and Parodies
Tags: good books, reading, what do you do all day?
Category Children's Books, Reading, What Do You Do All Day? |
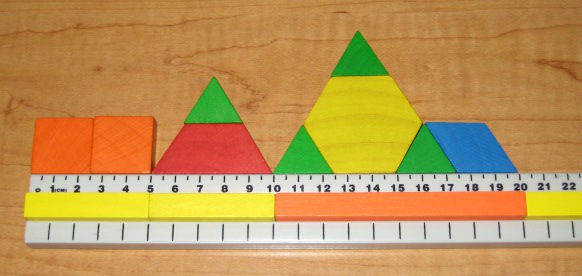
- Measuring lengths of Cuisenaire rods and pattern blocks using a Cuisenaire rod track
Earlier this week, I found some old notes I had written about playing with pattern blocks and Cuisenaire rods when Ben was about seven years old. We had been stacking the pattern blocks into towers and measuring them with the Cuisenaire rods. While doing this, we made some interesting comparisons. Here’s what I wrote:
“While measuring the pattern blocks, we noticed that they’re 1 cm thick, just like the Cuisenaire rods, and therefore can be measured using the rods or a meter stick. The lengths of the pattern block sides are either 2 1/2 cm or 5 cm long, depending on the shape. The sides of the orange squares and green triangles are 2 1/2 cm long, but one side of the red trapezoid is 5 cm long.”

- Stacking and lining up rods and blocks to measure and compare them
I had forgotten all about these details until now. I’m glad I had those notes to remind myself about the fun we had exploring these materials and making these observations.
Tags: connections, cuisenaire rods, mathematics, pattern blocks, what do you do all day?
Category Connections, Cuisenaire Rods, For the Love of Math, Pattern Blocks |

Designing and playing with wooden blocks of all kinds can be lots of fun. Sometimes it’s nice to use a frame to give yourself a specific space in which to work. A wooden serving tray with raised edges works well as a frame for playing with square color tiles, Cuisenaire rods, or pattern blocks.

Small cardboard boxes with low edges are good for Cuisenaire rods and color tiles.
A hexagonal plant stand with raised edges works as a great frame for pattern blocks.

Tags: art, color tiles, cuisenaire rods, mathematics, pattern blocks
Category Art, Cuisenaire Rods, For the Love of Math, Pattern Blocks, Square Color Tiles |


























































